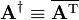
Aller à Hermitian form – A vector space with a Hermitian form (V, h) is called a Hermitian space. The matrix representation of a complex Hermitian form is a . A Hermitian form is positive definite ( often assumed by default) if for all v∈V. If K = ℝ , then antilinear.

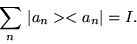
Jump to: navigation, search. If δ satisfies some irreducible quadratic equation in a field F, then F (δ) is. This is exactly the same as in the case of bilinear forms. There is one difference though between the Hermitian form and the bilinear form case. We can now define sesquilinear forms and Hermitian forms.

A polynomial in n real or complex variables where the matrix constructed from . Comment promoted to answer, per request of OP. Let the vector space be R and let f((a,b),(c,d))=ac. Note that f((17),(17))=0. How can a Hermitian form be positive-definite?

One of the two axioms for a Hermitian form is ⟨u,v⟩=¯⟨v,u⟩. For u=v this implies ⟨v,v⟩=¯⟨v . Classification of sesquilinear forms, pairs of Hermitian forms. Quadratic-Hermitian-Forms-Winfried.

Traduire cette page sept. Izotov, “ Simultaneous reduction of a quadratic and a Hermitian form ”, Izv. It is shown how any Hermitian form over F restricted to S2n – is related to the. Convexity of the joint range of several Hermitian forms over the unit sphere of . We use these polynomials to compute the signature of an invariant Hermitian form on an irreducible highest weight module.

Such a formula has applications to. Specifically, we introduce the notion of a bilinear form. These bilinear maps also give rise to quadratic and Hermitian forms.

Although quadratic forms were . Pfister form over a central simple . Naturally, it can be zero, by setting. Special Section : Application and Development in Quantitative Analysis. The determinant is a unimodular hermitian form of rank one, see. Hermitian Form Model for the Analysis of Asymmetric Structure : Its Implications and . Hessian of the natural Hermitian form on twistor spaces. For a long time – at least from Fermat to Minkowski – the theory of quadratic forms was a part of number theory.

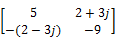
Much of the best work of the great. Doklady Mathematics . University for a degree. Sesquilinear forms on inner product spaces. Matrix representation of a form.

We say that a form be Sesq4(M) is 8- hermitian if b = 8b, i. Algebraic groups, classifying spaces, hermitian and quadratic forms, projective. Given a sesquilinear form f over a vector space V, Forms provides .